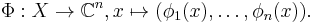Goldstine theorem
In functional analysis, a branch of mathematics, the Goldstine theorem, named after Herman Goldstine, asserts that the image of the closed unit ball 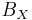 of a Banach space
of a Banach space  under the canonical imbedding into the closed unit ball
under the canonical imbedding into the closed unit ball 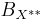 of the bidual space
of the bidual space 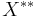 is weakly*-dense.
is weakly*-dense.
Proof
Given an 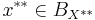 , a tuple
, a tuple  of linearly independent elements of
of linearly independent elements of 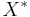 and a
and a 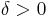 we shall find an
we shall find an  such that
such that 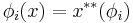 for every
for every 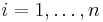 .
.
If the requirement 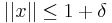 is dropped, the existence of such an
is dropped, the existence of such an  follows from the surjectivity of
follows from the surjectivity of
Let now 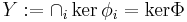 . Every element of
. Every element of 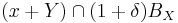 has the required property, so that it suffices to show that the latter set is not empty.
has the required property, so that it suffices to show that the latter set is not empty.
Assume that it is empty. Then 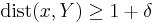 and by the Hahn-Banach theorem there exists a linear form
and by the Hahn-Banach theorem there exists a linear form 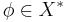 such that
such that 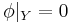 ,
, 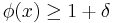 and
and  . Then
. Then 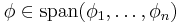 and therefore
and therefore
which is a contradiction.